Month: October 2021

We had a wonderful and varied collection of submissions! Every attendee of the exhibit was given the chance to vote on their favorites in various categories. Every submission was picked to be the favorite of at least one person, and the voting was very close in most categories. The submissions receiving the most votes in each category are listed below.

Grand Math-erpiece: Word Mandala, by Nancy Cleaver

This entry also won the Most Thought-Provoking category.

Word Mandalas are my explorations of integrating verbal and visual qualities of an idea into one expression.
All the lines form letters, joining one another in sectors of the circle.
In some mandalas, the letters become “grout” lines surrounding the colorful “mosaic” spaces between them. The letters organize the mandalas, then fade into the whole design.
A mandala (mahn-doll’-ah) is “an integrated structure organized around a unifying center”. Every circle, spiral, or sphere is a mandala; so are atoms, cells, planets and their orbits, and many plant structures. We use mandala forms in math, music, literature, religion, and visual arts. Once you begin looking for mandalas, you see and experience them everywhere.
If you would like to search for the word, relax into the image, and let the letters appear, not necessarily from left to right. You will be engaging your whole brain this way. Some are easier to find than others, and of course, you can simply enjoy the work visually.

Nancy Cleaver

Best Children’s: Adele McNamara, aged 10

Best Bucknell Student Entry (tied): Miriam Vollmayr-Lee and Peter (Tianzhu) Liu ’22

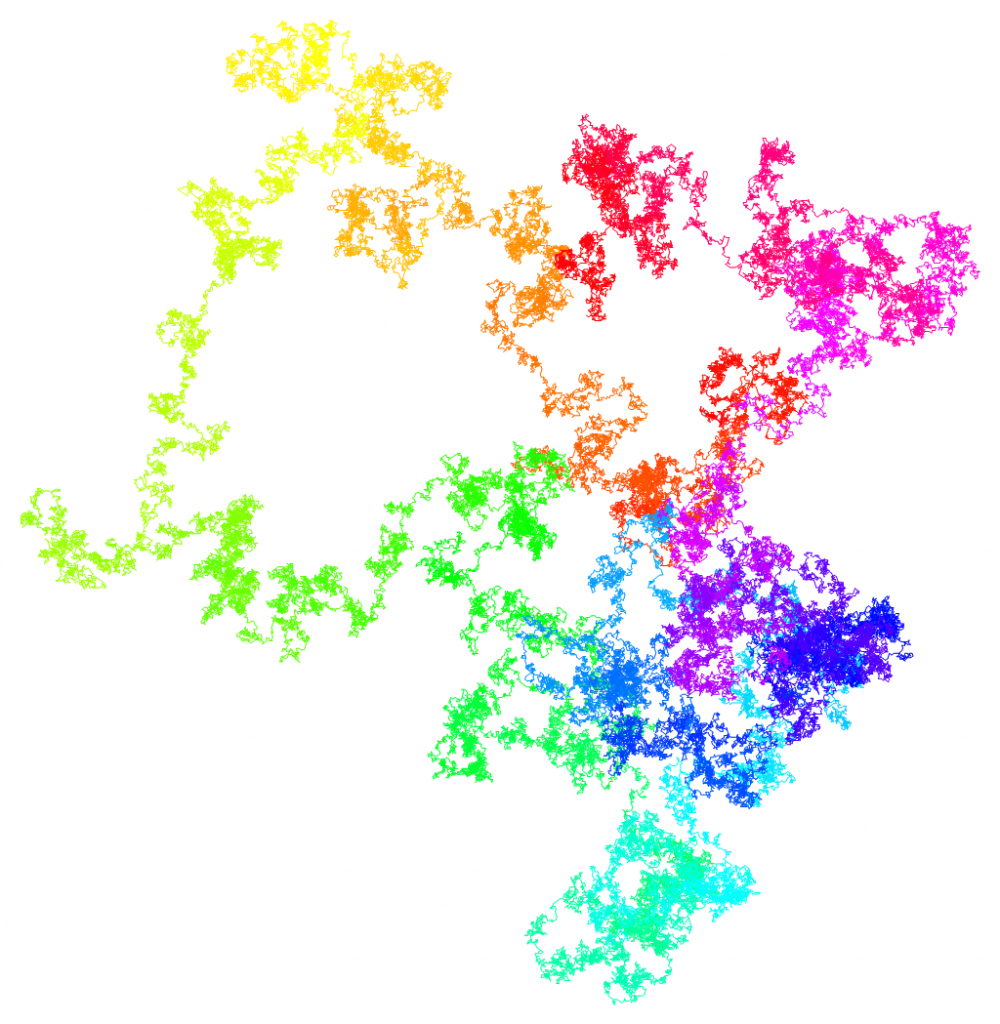
Mathematically Most Intriguing: Yang Hong ’23

Graphitization of the decimal form of π (up to 100000 digits after the decimal point).

The algorithm: each digit is transferred to a unit vector with a direction from 0π/5 to 9π/5. The color is representing the relative position (starting from red and ending at magenta in the sequence of rainbow color), and also making them artful.

Most attractive: Erica Merriett

Blackwork Embroidery
Pattern: “Just Birds” by Clare Ardali (peppermintpurple.com)

Blackwork embroidery is recognized by its geometric designs that often using repeating floral, star and lattice patterns to fill the inside of a larger shape. While traditional blackwork involves a black thread being stitched onto a white linen or cotton (which may or may not feature accent colors or tones), today the term “blackwork” is more commonly used to describe the technique rather than the use of black thread – so it still uses the delicate geometric designs, but can be stitched in any color.

Blackwork dates back to before the 16th century, and was popularized in England by Henry VII’s first wife, Catherine of Aragon, who brought blackwork clothing with her from Spain. This is why it is sometimes known as Spanish blackwork.

Most Fun: Jiawen (Jessica) Zhao ’22

Come enjoy the entries to the Mathy Art Contest and vote on your favorite in several categories!

Thursday 10/21 at 11:30am-noon and 1:10-1:40pm.
Great Room, Hildreth-Mirza Hall.
All are welcome.

This event is held in conjunction with the 12:10-1:10 talk in the same location:
“I Can’t Believe it’s (Knot) Math: Invariants and the Kauffman X Polynomial”
by Prof. Zach Cline.
Staying/Coming early for the talk is optional but encouraged

Student Colloquium:

Presented by the Mathematics Department Student Colloquium Series.

Great Room, Hildreth-Mirza Hall

Pizza served starting at 11:40; the Talk starts at 12:10pm.

Title: I Can’t Believe it’s (Knot) Math: Invariants and the Kauffman X Polynomial

Presenter: Zach Cline, Department of Mathematics, Bucknell University

Abstract: Once studied by mathematicians for their presumed connections to atoms, knots are now studied in their own right, and their study has revealed deep connections to other fields, like hyperbolic geometry and quantum field theory. They have also been used more pragmatically to study knotting phenomena in DNA. In this talk, we will define a few basic notions from knot theory, introduce the concept of invariants, which is the primary source of connections to other fields, and derive a particular invariant known as the Kauffman X Polynomial.

Mathy Art Contest Exhibit and Voting

Come enjoy the entries to the Mathy Art Contest and vote on your favorite in several categories!

Before and after the talk: 11:30am-noon and 1:10-1:40pm